13.14.2 Ho and Lee Model

Ho and Lee extended the Merton Model to fit a given initial yield curve perfectly in a discrete-time framework. In the Ho and Lee Model case, we assume that the short rate of interest is again the single stochastic factor driving movements in the yield curve. Instead of assuming that the short rate r is a random walk, however, we assume that it has a time-dependent drift term θ(t) :

Figure 13-9 Ho and Lee Model Formula

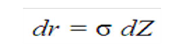
Description of formula to calculate the dr for Ho and Lee Model follows:

As before, Z represents a standard Wiener process with mean zero and standard deviation of 1.

By applying the no-arbitrage condition, the function q(t) is chosen such that the theoretical zero-coupon yield to maturity and the actual zero-coupon yield are the same. The function q(t) is the plug that makes the model fit and corrects for model error, which would otherwise cause the model to give implausible results. Because any functional form for yields can be adapted to fit a yield curve precisely, it is critical, in examining any model for plausibility, to minimize the impact of this extension term. The reason for this is that the extension term itself contains no economic content.

In the case of the Ho and Lee model, the underlying model would otherwise cause interest rates to sink to negative infinity, just as in the Merton model. The extension term's magnitude, therefore, must offset the negative interest zero-coupon bond yields that would otherwise be predicted by the model. As maturities get infinitely long, the magnitude of the extension term will become infinite in size. This is a significant cause for concern, even in the extended form of the model.